УДК 504.03
M. S. Safonova V. A. Tabunshchik R. V. Gorbunov T. Y. Gorbunova	Radiation balance of low-mountain sub-Mediterranean forest landscapes (on the example of the Karadag Nature Reserve)
	A.O. Kovalevsky Institute of Biology of the Southern Seas of RAS, Sevastopol, Sevastopole-mail:malashina@ibss-ras.ru

Abstract. New quantitative data on elements of the radiation balance of the downy-oak forests of the southeastern Crimea on the example of the Karadag Nature Reserve are presented. Elements of the short-wave part of the radiation balance of the crown surface and the long-wave part in the downy-oak forests are calculated. For the first time, the value of the solar radiation input to the crown surface in the downy-oak and juniper forests for the forests of the South-Eastern Crimea was calculated together with the values of the solar radiation transmission under the canopy of the forest Spatial models of transmission of aggregate solar radiation by the crowns of downy oak forestsare constructed. The amount of transmission of solar radiation is shown to be greatly influenced by the shape and density of leaves, as well as the shape of branches. The obtained data show a complex spatial-temporal distribution of solar radiation on the crown surface and in the under-crown space within the downy-oak forests.

Keywords: downy-oak forest; radiation balance; Karadag; Crimean Peninsula

Introduction

The importance of studying the radiation balance and solar radiation intake is due to their leading energetic role in forming the structure and ensuring the functioning of ecosystems. It is the redistribution of the radiation balance in the landscape that determines the spatial and vertical differentiation of the ecosystem structure.

The theoretical foundations for studying the radiation balance of an ecoregion territory are established in the works (Budyko, 1961; Berlyand, 1956; Budyko, 1956). However, the methods described in (Budyko, 1961; Berlyand, 1956) relied on a number of meteorological parameters that did not consider the features of the plant community in the studied area. The theoretical foundations underlying the study of the radiation balance of forests, which take the influence of vegetation into account, are described in the works (Reifsnyder, 1968; Dzerdzeevskii, 1963; Moore, 1976; Bergen, 1969; Lull, Reigner, 1967). D. P. Akimova (Akimova, 1972), V. A. Alekseev (Alekseev, 1975), N. N. Vygodskaya (Vygodskaya, 1967, 1977), N. V. Zukert et al. (1967), N. A. Bityukov (Bityukov, 2012), I. S. Ugarov (Ugarov, 2018) also studied the radiation balance of forest communities.

However, for the ecoregion of the forests of the Crimean Peninsula as a whole, there is rather scant information about the values of incoming radiation and the radiation balance (Korsakova, 2015; Kondratyev, Manolova, 1960; Schaab et al., 1999). An example of the calculation and description of the radiation balance in Crimea at the regional and local levels appears in the works of R. V. Gorbunov et al. (2020a, 2020b), which were carried out for landscapes of oak, beech and pine forests, as well as in the work of V.O. Smirnov (Smirnov, 2012; Smirnov, 2016). E. I. Yergina and co-authors (Ergina et al., 2017) indicate that they have constructed a map of the radiation balance of the territory of the Crimean Peninsula; however, the map itself is not presented. L. Y. Garkusha and L. A. Bagrova (Garkusha, Bagrova, 2012) calculate an average radiation balance of 55.4 kcal/cm2 per year within the oak forests of the northern macroslope of the Crimean Mountains. V. V. Antyufeev (Antyufeev, 1988) provides radiation balance calculations and cartograms for the territory of the western and central parts of mountainous Crimea, which however exclude the area studied in the present work. Presenting radiation balance values in the landscape areas of the mountainous Crimea, V. V. Antyufeev (Antyufeev, 1988) indicates that on Karadag they retain 2365 MJ/m2.

Local studies of solar radiation have also been conducted in the Nikitsky Botanical Garden (Korsakova, 2015; Fursa et al., 2006). The annual value of direct solar radiation in this area is 2613 MJ/m2; scattered – 1792 MJ/m2; total – 4405 MJ/m2 (Korsakova, 2015). V. O. Smirnov (Smirnov, 2012; Smirnov, 2016) investigated the values of the radiation balance at the local level on the territory of the Cape Martyan Reserve. Some data on the radiation balance and its components for Crimea are given in the works of N. I. Goisa (Goisa, 1961; Goisa, 1964), A. A. Borisov (Borisov, 1963), E. P. Barashkova (Barashkova, 1961), E. M. Chumakova (Chumakova, 1947), S. I. Sivkov (Sivkov, 1940), as well as in a few climatic atlases (Ukrainian SSR. Atlas…, 1968; National Atlas of Ukraine, 2007), and various other publications.

However, not enough attention has been paid to an integrated approach in studying the intake of solar radiation or the radiation balance of the local level, affecting certain types of plant communities. The radiation balance of various types of ecosystems and landscapes has recently received much attention internationally, including in China (Song et al., 2017; Wu et al., 2007; Wang et al., 2022), Russia (Dudorova, Belan, 2015; Kharyutkina et al., 2012; Li et al., 2013), the USA (Parker et al., 2004), Brazil (Giambelluca et al., 1997; Bastable et al., 1993), Thailand (Giambelluca et al., 1999), Madagascar (Ghimire et al., 2022), Israel (Stanhill et al., 1973), and other countries of the world.

Although a large number of studies (Schaab et al., 1999; Seyednasrollah et al., 2019; Todt et al., 2019; Fu, Rich, 2002; Isabelle et al., 2018; Schleppi, Paquette, 2017; Terez, Terez, 2002; Webster et al., 2017; Olpenda et al., 2018) describe methods for studying incoming solar radiation in forest communities, these are mainly focused on specific cases: consideration of individual spectra (visible radiation, illumination), the influence of trees on snow melting, etc. Nevertheless, the authors themselves emphasise the influence of various types of forests, including the formation of microenvironmental conditions, which affects the dynamics of the forest, ecosystem processes and the composition of the habitat.

Will Simonson and co-authors (Simonson et al., 2014) consider the influence of the topographic position on the distribution of tree species, which entails varying density and crown height parameters. Thus, the characteristics of the vegetation cover affect the intake of solar radiation and the amount of undergrowth. Solar radiation is also considered as a condition for the dissemination of particular tree species (Yilmaz et al., 2012). According to the studies of the mountain forests of Turkey, the distribution of forest species from the smallest amount of total solar radiation to the largest has the following form: Beech-Spruce-Fir-Oak-Pine-Cedar-Juniper. Based on work carried out in Italian oak forests, Gertrud Schaab’s study (Schaab et al., 1999) focused on the distribution of shaded canopies and their change in time and space in complex terrain (crown relief/dissected terrain). A. Liakatas (Liakatas et al., 2006) considers the amount of solar radiation absorbed, reflected or transmitted by vegetation depending on the distribution of radiation and the properties of vegetation cover. It was shown that the natural oak forest on the territory of Greece absorbs about 70% of radiation during the growing season, while the remaining part can be attributed to reflection and transmission. In winter, the transmission of solar radiation is over 40%.

The main ridge of the Crimean Mountains forms a circulation barrier that stops cold northern air masses and delays warm southern ones; thus, a sub-Mediterranean (or semi-subtropical) climate has formed in the territory of southeastern Crimea with warm winters, maximum precipitation during the cold period of the year (late autumn–winter) and hot, mostly dry summers. Due to these conditions, downy-oak formations appear on the border of their range in the territory of the southeastern Crimea along with an admixture of Jerusalem thorn, smoke bush, and Oriental hornbeam. These are represented from the coast to an elevation of 300–350 m (Bokov, 2001).

An investigation of the radiation balance of downy-oak forests located at the furthest extent of their existence is an important task, since it allows us to understand their individual organizational patterns. Depending on the nature of the plant community growing in a particular territory, a crown relief that is distinct from that of the Earth’s surface redistributes the flow of light and energy along its vertical structure. The most illustrative example of this phenomenon is given by forest communities whose crown surface is located a few meters above the surface, forming a sub-crown space with its own functioning features.

The purpose of this study is to determine the influence of forest vegetation on the elements of the radiation balance. To achieve this goal, a series of tasks were diligently pursued: the measurements of the main climatic indicators within both open expanses within the downy oak forest’s growth zone and in the forest itself; the influence of the crown relief on solar radiation inflows during the day was revealed; the constituent elements of radiation balance were calculated and a spatial-temporal analysis of their changes was carried out.

Materials and methods

Research Area

For describing the features of the radiation balance and intake of solar radiation in the forest ecosystems of southeastern Crimea, downy-oak forests were selected as key sites.

The downy-oak forest is located on the territory of the permanent study area of the Karadag Nature Reserve, which is situated on the slope of the eastern aspect of the Besh-Tash ridge. This location is typical for the Crimean Peninsula, a significant part of whose mountainous part is occupied by downy-oak forests growing in the lower forest belt of the southern coast and northern foothills on slopes up to 45° steepness. In general, this species occupies about 20% of the forests of mountainous Crimea.

The section with a downy-oak forest is located on a gentle slope of the eastern aspect (Fig. 1), represented by one arborescent stratum of downy oak (Quercus pubescens) at an average height of 5 meters and trunk diameters of 20 cm, along with oriental hornbeam (Carpinus orientalis) at a height of 3.5 meters. The undergrowth of young oak trees up to 30 cm high is found throughout the study area.

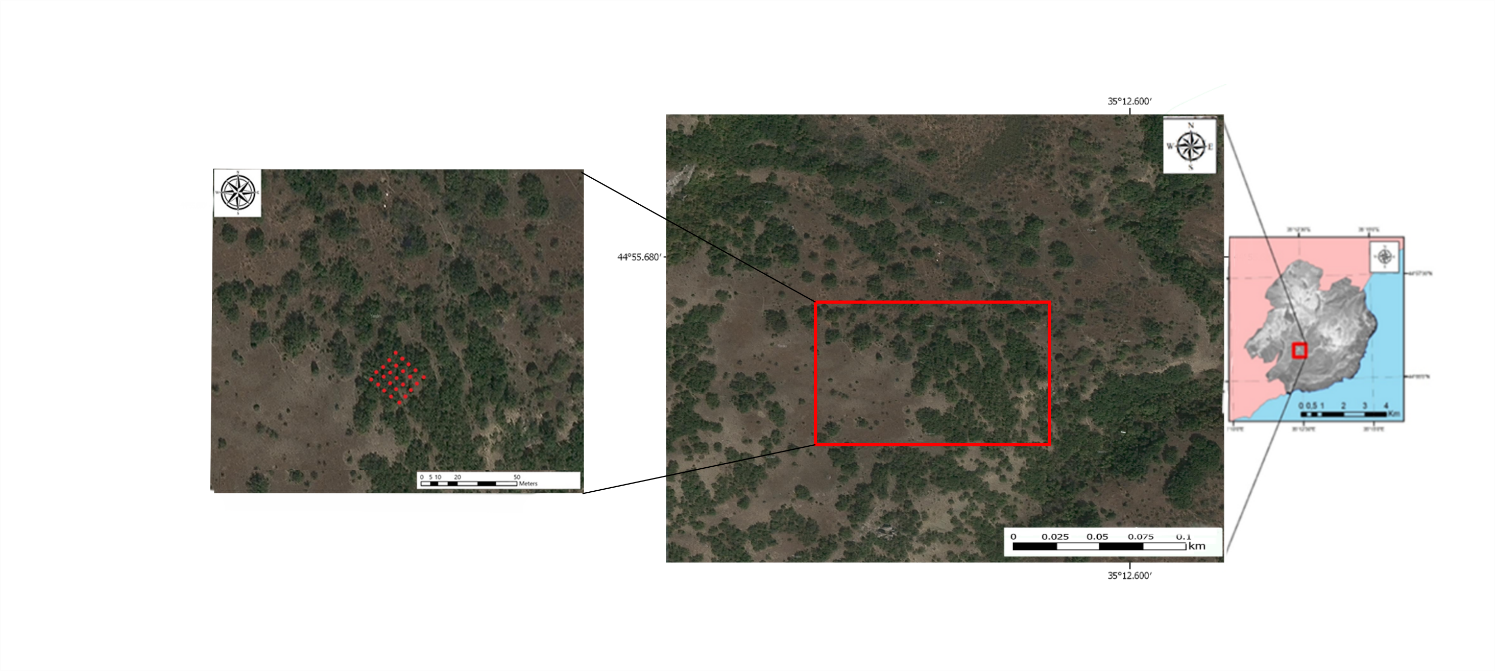
Figure 1. Location of the downy-oak forest site and network of measurement points and location of the monitoring weather station in the downy oak forest

The shrub layer is represented by smoke tree (Cotinus coggygria), cornelian cherry (Cornus mas) and Jerusalem thorn (Paliurus spina-christi).

The following herbs are presented: burning bush (Dictamnus gymnostylis), campion (Silene densiflora), voronova volodushka (Bupleurum woronowii), knapweed (Centaurea caprina Steven), Centaurea × panciciana Prod. (family Asteraceae), Callea Fescue (Festuca callieri).

Research Methods

Sites for measuring the main meteorological parameters of both selected communities were organised. Each site consists of two parts: an open area in the forest growth zone, which ensures the fixation of values characteristic of this ecosystem without the influence of woody vegetation, and a typical community forest area, in which a number of measurements are duplicated in order to determine the conditions of the landscape and the influence of vegetation on the distribution of matter and energy flows.

The research was carried out on the basis of data from the Davis Vantage Pro2 meteorological monitoring station installed on the territory of the landscape and in the open part of the environmental permanent study area, which displays all meteorological parameters in real time, while the sensor control console records the measured values hourly. Long-term meteorological parameter values for a given area were obtained using the MERRA reanalysis database (MERRA-2 meteorological re-analysis. 2023). After obtaining the amount of incoming solar radiation based on these values, the radiation balance for the community as a whole was calculated.

Measurements in forest communities were carried out within typical forest plots measuring 20×20 m according to a regular grid of points (Fig. 1) located every 5 m.

At each point, the transmission of solar radiation by the crowns of trees was measured using the DT-1309 digital light meter at a height of 1 m above the soil surface according to the method described by V. O. Smirnov (Smirnov, 2016), according to which the measured values under the tree canopy are compared with the value in the open area. As a result, the percentage of solar radiation transmission by the crowns of downy-oak forests was calculated. In order to describe the temporal variability of this parameter, measurements were taken at 9:00, 12:00, 15:00 and 17:00 hours in clear windless weather.

For a more complete description of the condition of the crowns of trees and the features of their retention of incoming radiation, our measurements were carried out in the summer growing season.

At each point, the values of the height of trees and undergrowth were obtained along with the proximity of crowns and degree of illumination. After comparing the obtained values with the value of illumination in the open area, the ratio was used to calculate the value of the total solar radiation penetrating to the ground surface under the crowns of trees.

In order to study the temperature and humidity regimes under the forest canopy, data loggers of the TR series were installed. These were used to record the hourly temperature and humidity of the air at a height of 0.5 m and 2 m above the soil surface. The obtained data were used to calculate the magnitude of the effective radiation of the surface of the soil litter within the downy-oak forest.

The height of the crown relief was obtained using the DJI P4 Multispectral quadcopter. The footage was processed using the Pix4D software to create an orthophotomap representing information about the values of the heights of the crown relief. The previously constructed digital model of the fairly heterogeneous and complex surface of the crowns was used to calculate the steepness and aspect of the slopes of the crown relief in the downy-oak forest, as well as to create a model of the total solar radiation input to the surface of the crowns under cloudless skies. The distribution of solar radiation according to the morphometric characteristics of the crown relief was simulated using a standard set of ArcGIS tools. Based on the data of the crown relief and the geographical coordinates of the study area, the distribution of incoming solar radiation is modeled, taking into account the position and angle of inclination of the sun relative to the surface and the features of the relief.

The radiation balance calculation (B) was carried out according to M.I. Budyko’s formula (Budyko, 1961):

B = (Q − R) − Ee, (1)

where Q – total solar radiation; R – reflected solar radiation; Ee – effective radiation.

The reflected radiation was calculated by the formula:

R = A ∙ Q, (2)

where A – albedo of the Earth’s surface.

The albedo was determined according to the data given in the work of V. V. Rakhmanov (Rakhmanov, 1984). The difference between the total and reflected radiation gives the amount of absorbed short-wave radiation – or short-wave radiation balance.

Calculations of the long-wave radiation balance elements were carried out on the basis of data obtained using data loggers. Calculations were carried out on the basis of D. Brunt’s formula (Brunt, 1932) using values obtained during the measurement period of the transmission of solar radiation by crowns. Since the temperature and humidity measurements were carried out at one typical point, the values of the elements of the long-wave part of the radiation balance can be calculated as average values for sections of downy-oak forests.

The effective radiation of the Earth ‘s surface was determined by the formula:

Ee = Es − δEa, (3)

where Es – thermal radiation flux of the underlying surface directed towards the atmosphere; Ea – anti-atmospheric emission; δ – relative emissivity of the surface.

The thermal radiation flux of the underlying surface directed towards the atmosphere is determined by the formula:

Es = δσT4, (4)

where σ – Stefan–Boltzmann constant; T – air temperature.

The atmospheric counter radiation was determined by the formula of D. Brent:

Ea = δσT4 (a ′ + b√e), (5)

where a’ = 1 − a; a and b – empirical constants according to M. E. Berlyand (a = 0.39; b = 0.058) (Berlyand, 1956); e – partial pressure of water vapour.

Results

Radiation balance within an open area

When considering the radiation balance of an open area, it is possible to trace the main trends of its changes in intra- and inter-annual dynamics within the limits of the downy-oak forests. Concerning the data for the last 10 years, there are significant fluctuations in the values of the annual balance between a minimum in 2021 of 855 MJ/m2 and maximum in 2017 of 1126 MJ/m2. Thus, the amplitude of the values of the annual radiation balance reaches 271 MJ/m2 (Fig. 2). While the trend line is characterised by an increase in the average long-term value of the radiation balance, in recent years there has been a decrease in the indicator under consideration.

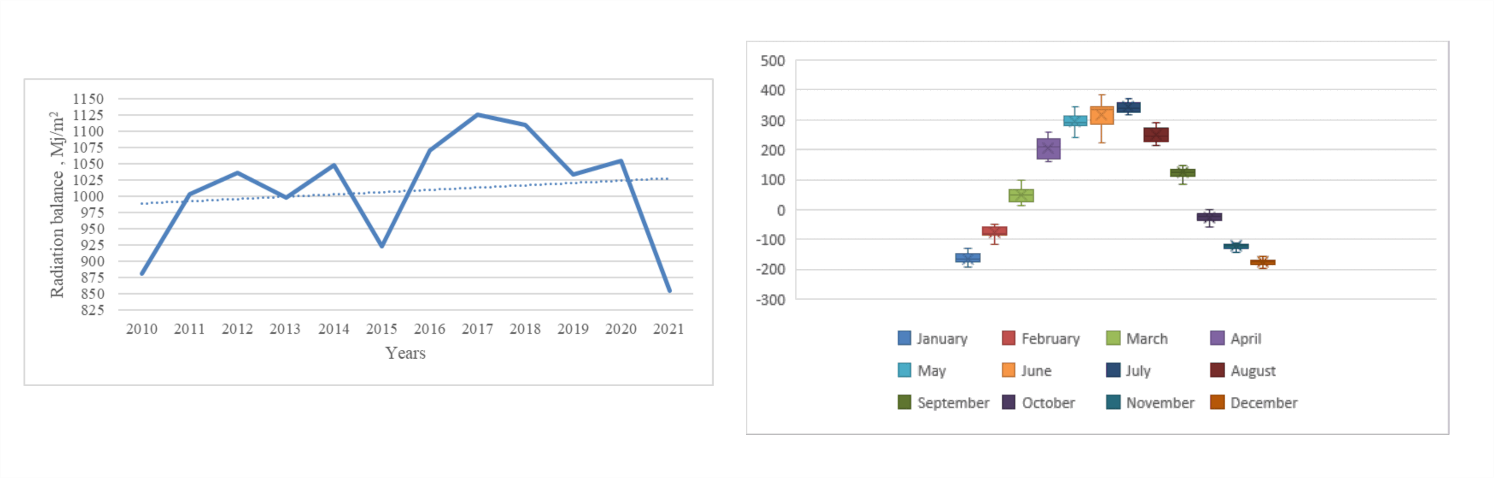
Figure 2. Values of the annual radiation balance of the permanent study area, MJ/m2. (left) and intra-annual average radiation balance values by month, MJ/m2. (right)

When considering the intra-annual dynamics, two periods are naturally distinguished (Fig. 2): a positive balance in the summer period from March to October, and a negative balance in winter, from November to February. The lowest annual average value (−176.3 MJ/m2) is minimal for December, while the maximum value of the radiation balance is observed in July (341.8 MJ/m2). The relatively smooth transition from the lowest value to the highest and back occurs cyclically with the change of seasons.

Analysing the radiation balance indicators by month for different years, it is noticeable that the greatest variation in values occurs during the warm period of the year, while in winter, the differences are minimal (Fig. 2). The greatest amplitude of changes in values is observed in April and June. Given the weak winter fluctuations, it can be assumed that the variability of the values of the radiation balance of the summer months is reflected in the fluctuations of the values of the annual balance.

Radiation balance within the downy-oak forest

The radiation balance values described above relate to an open horizontal area without taking vegetation cover into account. However, the downy-oak forest forms its own crown relief, which delays a certain amount of incoming solar radiation on its surface, as well as transmitting part of it into the under-canopy space. The degree of transmission of solar radiation by the crowns depends on the closeness of the crowns and their density, including the presence of gaps inside the crown (Table 1). Deciduous trees are characterised by seasonal changes in the amount of foliage in the crown, up to its complete absence in winter. For a more complete description of the condition of the crowns of trees and the features of their retention of incoming radiation, our measurements were carried out in the summer growing season, i.e., the growing season and the state of maximum crown development.

Table 1

Main parameters of the arborescent stratum at points in the downy-oak forest.

№ Poin	Tree height, m	Crown density, %	Crown closeness, %
1	5,5	80	95
2	5,5	60	80
3	6	60	85
4	5,5	60	90
5	5,5	30	50
6	5,5	70	50
7	4	100	30
8	2	90	40
9	3,5	100	50
10	3	95	50
11	5,5	80	45
12	5	100	10
13	3,5	30	30
14	3,5	70	50
15	3,5	50	20
16	5,5	80	30
17	4,5	90	30
18	5,5	60	60
19	1,7	70	60
20	3,5	100	50
21	3,5	100	100
22	0,3	0	0
23	3,5	40	10
24	1,5	20	2
25	3	80	10

Crown closure is understood as the fraction of the Earth’s surface area occupied by crown projections. However, this indicator does not reflect the actual area of the Earth’s surface that is shaded by the forest canopy. Therefore, for a more accurate description of the crown canopy, its density is also considered.

It is also worth noting the uneven receipt of solar radiation under the canopy of the forest, which can be explained by the presence of areas not covered with crowns, as well as the spatio-temporal distribution of shadow masks. The amount of incoming solar radiation to a specific point on the surface depends on its orientation relative to the direction of the sun’s rays, i.e., it is determined by the steepness and aspect of the slopes of the crown relief (Fig. 3).

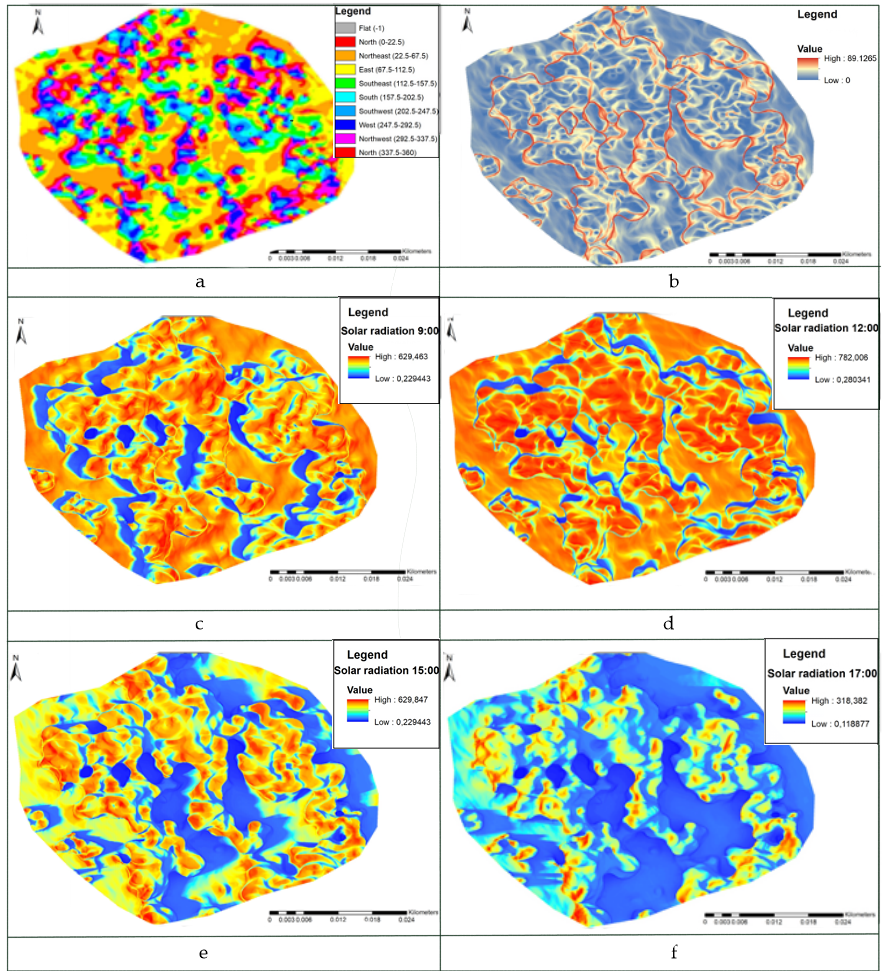
Figure 3. Characteristics of the crown relief in the downy oak forest: aspect of the slopes of the crown relief (a); steepness of the crown relief, ° (b); total solar radiation received by the crown surface at 9:00, W/m2 (c); total solar radiation received by the crown surface at 12:00, W/m2 (d); total solar radiation received by the crown surface at 15:00, W/m2 (e); total solar radiation received by the crown surface at 17:00, W/m2 (f).

The total solar radiation received on the crown surface at different times of the day based on the obtained models of the steepness and aspect of the slopes of the crown relief is shown in Table 2.

Table 2

Estimated amount of total solar radiation received on July 17 (date of field measurements), depending on the steepness and aspect of the slopes of the crown relief of the downy oak forest, W/m².

Aspect	Steepness
	0–3°	3-8°	8–15°	15–30°	30–45°	>45°
	9:00
N	474.67	451.76	468.18	401.19	283.67	71.90
NE	489.01	504.42	507.66	512.77	495.98	387.28
E	506.82	525.67	540.86	572.54	593.33	337.65
SE	503.63	518.61	550.90	578.77	601.29	552.61
S	499.55	497.89	505.78	499.89	416.27	390.14
SW	493.98	466.57	43.85	382.94	282.26	53.99
W	490.21	456.77	411.20	129.25	177.62	54.22
NW	483.93	428.46	430.45	328.88	202.02	49.90
12:00
N	697,01	663,83	671,39	554,08	368,53	85,18
NE	711,23	702,20	667,94	558,26	469,91	168,05
E	730,70	727,65	701,65	647,53	592,47	275,52
SE	734,02	738,42	755,06	734.38	707.93	550.23
S	737.96	743.78	763,37	773.53	683.03	650.44
SW	737.43	731.01	672.14	735.73	682.88	482.94
W	730.75	717.54	705.88	605.50	567.68	279.01
NW	721.08	505.15	687.15	599.68	473.87	60.97
15:00
N	474,65	299,71	468,06	401,07	48,89	69,72
NE	475,59	456,40	413,34	319,29	121,19	78,52
E	485,78	462,68	407,84	269,32	91,98	36,71
SE	490,58	474,51	458,10	379.02	308.43	84.64
S	499.55	497.89	505,78	499.89	45.73	390.14
SW	506.11	513.67	234.46	577.62	586.73	508.82
W	500.61	514.92	544.42	131.34	586.71	517.26
NW	496.56	74.06	520.40	521.43	495.79	317.89
17:00
N	50,85	41,70	178,74	128,82	25,33	36,15
NE	166,93	110,69	51,44	77,94	46,09	40,70
E	169,98	154,33	120,39	43,16	47,64	19,02
SE	171,71	157,19	139,62	49.86	51.53	41.90
S	177.00	117.63	168,80	154.61	23.69	56.65
SW	182.28	185.85	22.72	197.31	235.39	59.29
W	179.92	53.62	152.40	27.77	37.10	301.15
NW	180.17	38.37	210.02	237.01	254.26	25.86
The whole day
N	3958,27	5317,05	5111,05	4292,32	2059,90	789,53
NE	5344.72	5254.15	4911.59	4572.83	3708.03	2241.04
E	5508.06	5470.72	5084.35	4710.69	3815.68	1854.26
SE	5365.57	5510.57	5606,69	5241.09	5117.60	3984.59
S	5543.85	5544.62	5632.39	5573.38	3723.64	4360.25
SW	5548.32	5304.56	2780.25	1827.29	4877.03	3268.02
W	5496.73	5112.14	5195.77	3203.56	4406.58	147.75
NW	5493.69	5406.05	5156.53	4670.29	3777.11	1330.60

As can be seen from Figure 3 and Table 2, there is a characteristic distribution of incoming solar radiation along the slopes of the crown relief located in the northern hemisphere, where the largest amount of it enters the slopes of the crown relief of the southern aspect, while the northern sleeps are to some degree shaded depending on the steepness of the slope of the crown relief. Approximately equal values of incoming solar radiation are observed on the slopes of the western and eastern crown aspect, the only difference being in the distribution of the specified amount over time.

On the slopes of the crown relief of the northern aspect, the greatest amount of incoming solar radiation is observed on the gentle slopes of the crown relief (0–3°); this value decreases gradually with increasing steepness. A sharp decrease in total solar radiation occurs when the steepness of the slopes of the crown relief increases by more than 30°. There is also an increase in the amount of total solar radiation at 15:00 and 17:00 on the slopes of the crown relief of medium steepness (8–15°). The maximum values for the slopes of the crown relief of the northern aspect are recorded on the gentle slopes (0–3°) at 12:00, while the values of the total solar radiation intake in the morning and evening hours are approximately the same.

In the morning, radiation values are slightly higher on the slopes of the southern aspect of the crown relief than on the northern ones; however, with an increase in steepness, a significant excess is observed. In general, the greatest amount of incoming total solar radiation is observed for any time of day on the gentle slopes of the crown relief, which gradually decreases with increasing steepness of the crown relief. The maximum values noted at 12:00 on the slopes under consideration are relative to the slopes of other aspects. The significantly lower values of total solar radiation at 17:00 relative to those recorded in the morning decrease sharply with a more than 30° increase in the steepness of the slopes of the crown relief.

The total solar radiation received by the slopes of the crown relief of the western aspect in the morning hours is marked by a sharp decline in the change in values with a slope steepness of more than 15°. A gradual decrease in values recorded for noon is characterised by a sharp decrease with an increase in steepness of more than 45°. At 15:00, the maximum values are noted relative to the slopes of the crown relief of other aspects, with minor fluctuations occurring during the day, but without significant decreases when the steepness changes. At 17:00, the solar radiation values are significantly lower than at other times of the day, with the highest values being recorded on extremely steep slopes of the crown relief (more than 45°).

The maximum values recorded on the slopes of the crown relief of the eastern aspect in the morning are fixed relative to the slopes of the crown relief of other aspects; here, a significant decrease in values is noted on extremely steep slopes of the crown relief with a steepness of more than 45°. A similar distribution of values along the steepness of the crown relief occurs during the daytime. The opposite situation develops in the evening (15:00 and 17:00), when the highest values are observed on the gentle slopes of the crown relief; when moving to the steep slopes (15-30°) of the crown relief, there is a sharp drop in the values of the total solar radiation intake.

By averaging the daily values of the total solar radiation intake on the surface of the slopes of the crown relief of different steepness, we can estimate its distribution as a whole depending on the aspect. Thus, the significant difference in the values of the slopes of the crown relief of the northern and southern aspects, in which the slopes of the northern aspect of the crown relief are mainly in the shade, is due to their location the northern hemisphere. Although the nature of the distribution of total solar radiation on the slopes of the crown relief of the western and eastern aspects are approximately equal, the increased total solar radiation received by the eastern – and, especially, southeastern – aspects of the slopes of the crown relief is most likely due to the orientation of the slope on which the area of the fluffy oak forest is located.

As with any actual surface, when incoming solar radiation enters the relief of the crowns, it is partially reflected from the surface. The amount of reflected radiation depends on the diffuse reflectivity of the surface, i.e., the albedo, whose average value for a deciduous forest is 18%. Thus, we obtain the following distribution of radiation balance elements for the crown surface (Table 3).

Table 3.

Values of elements of the radiation balance of the surface of the crown downy oak forest crowns, W/m².

Elements of the radiation balance			Time
			9:00	12:00	15:00	17:00
Downy-oak forest	Total	mean	463.83	590.18	332.26	97.60
		max	593.33	763.37	505.78	178.74
		min	71.90	85.18	36.71	19.02
	Reflected	mean	83.49	106.23	59.81	17.57
		max	106.80	137.41	91.04	32.17
		min	12.94	15.33	6.61	3.42
	Absorbed	mean	380.34	483.95	272.45	80.03
		max	486.53	625.96	414.74	146.57
		min	58.96	69.85	30.10	15.60

As with the total solar radiation, the reflected and absorbed values, while maintaining a regular dependence on the steepness and aspect of the slopes of the crown relief, are characterized by significant variations in both time and space. During daylight hours, the values of the radiation balance elements increase to a maximum at 12:00, and then start decreasing towards 17:00.

Here, it is important to note that the presented values would be correct if all incoming solar radiation was delayed by the crowns; however, under real conditions there is always a certain percentage of transmission under the canopy of the forest. Therefore, the values presented above are overstated. Thus, in order to describe the vertical structure of the distribution of energy flows in the downy-oak forest, the amount of solar radiation transmitted by the crowns was studied.

The spatial-temporal structure of the illumination of the subspecific space of the downy-oak forest is obtained via a regular grid of points (Fig. 4).

Figure 4 demonstrates the significant spatiotemporal heterogeneity of transmission of total solar radiation by tree crowns. In most cases, the amount of solar radiation received under the crown does not exceed 20% of the initial value, while values exceeding 40 and 50% of the transmission of solar radiation are recorded at the same observation points. As clearly shown by the presented scheme, each point has its own transmission time of the maximum and minimum amounts of radiation. This is explained by changes in the distribution of gaps and shadow masks in the canopy depending on the time of day and the Sun’s angle position. A striking example of such a change is observed at point No. 22, where the amount of solar radiation received during the first half of the day corresponds to an open area, while in the second half of the day it is in the shade.

Figure 4. Spatio-temporal structure of the proportion of transmission of total solar radiation by the forest canopy.

Earlier in (Gorbunov et al., 2019), the absence of a correlation between the transmission fraction and the characteristics of the vertical structure was described; based on this, the crown relief model was not taken into account when constructing the spatial structure of the total solar radiation transmission value at different times of the day (Fig. 5).

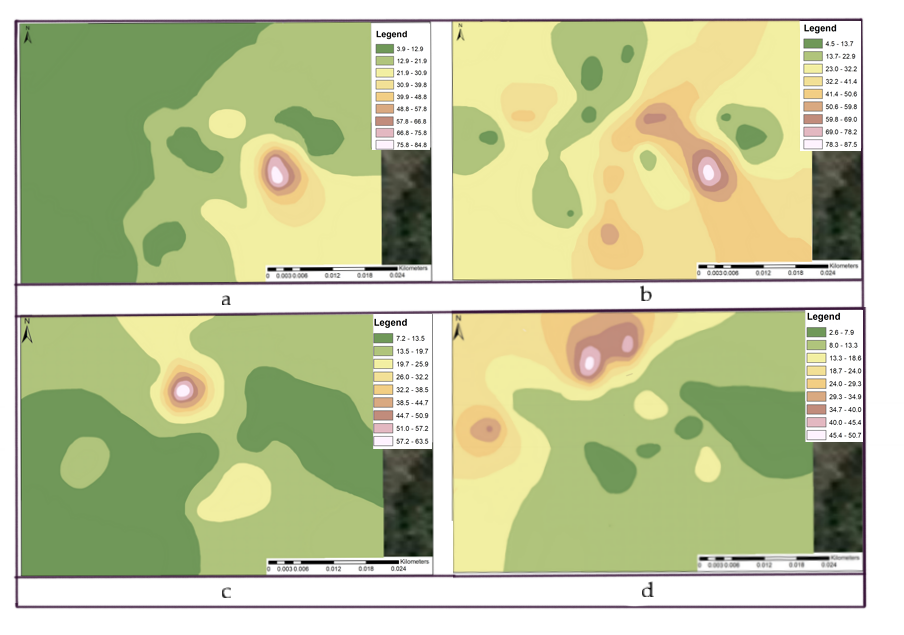
Figure 5. Spatial change in the proportion of transmission of total solar radiation by the canopy of a downy-oak forest, %: 09:00 (a); 12:00 (b); 15:00 (c); 17:00 (d).

When considering the spatial change in the transmission of total solar radiation, the forest canopy distinguishes areas with maximum transmission; these are open, i.e., not falling under the canopy of trees, or bordering open areas of the forest. However, since they are located inside the plant community, the neighbouring areas surrounding them have a significant impact on the processes of distribution of incoming energy occurring in them. In turn, open and semi-open areas also have an inverse effect. Thus, during daylight hours, conditions are formed in which the surrounding trees form a stable shadow on the neighbouring plot, while open areas allow total solar radiation to freely penetrate under the canopy of the forest.

After analysing the obtained values of the total solar radiation intake and the degree of transmission by the forest canopy, statistical values of the total solar radiation entering the subsurface space were obtained (Table 4).

Table 4.

Total solar radiation passed through the crowns of trees downy oak forest, W/m².

Total solar radiation		Time
		9:00	12:00	15:00	17:00
Downy-oak forest	mean	72.72	183.67	52.61	13.61
	max	463.83	590.18	268.77	56.35
	min	9.43	10.45	17.85	2.02

Solar radiation passing through the crowns of trees can also be divided into reflected and absorbed portions. However, the reflected short-wave radiation cannot be estimated at present due to the complexity and mosaicity of the surfaces under the canopy. Absorbed radiation is converted into a long-wave form of thermal radiation flux of the underlying surface that is directed into the atmosphere, as well as the atmosphere’s own thermal radiation onto the Earth’s surface. The effective radiation values are obtained based on these two values (Table 5).

Table 5.

Values of elements of the long-wave part of the radiation balance of the downy-oak forest during the measurement period, W/m².

Elements of the radiation balance		Time
		9:00	12:00	15:00	17:00
Downy-oak forest	Thermal radiation of the underlying surface	406.90	409.68	414.16	415.29
	Intrinsic thermal radiation of the atmosphere	334.12	335.65	323.88	316.35
	Effective radiation of the underlying surface(soil surface, leaf litter, grass layer)	82.80	84.10	100.00	108.44

Even without being able to calculate the short-wave part of the radiation of the radiation balance, a comparison of the values of Tables 4 and 5 shows that there is an excess of outgoing radiation over incoming radiation within the average values. The exception is the middle of the day, which accounts for the daily maximum of incoming total solar radiation. In addition, the presence of incoming radiation values significantly exceeding the effective radiation of the underlying surface is noted. This can be explained in terms of significant gaps in the crown of trees, which, in contrast to the negative radiation balance of the main part located in the shade of tree crowns, form a positive radiation balance of the forest’s under-canopy space. This distribution of values demonstrates the complex organisation and flow of the distribution of matter and energy fluxes in the downy-oak forest.

The new data on the radiation balance of downy-oak forests obtained as a result of the conducted research inform a better understanding of the processes occurring in the ecosystems of these forests.

The study is the first to be conducted taking into account the influence of the crown relief for the southeastern Crimea, as for the Crimean Peninsula as a whole. Nevertheless, similar studies should also be carried in the remaining seasons of the year, in order to identify inter-seasonal dynamics and establish changes in the radiation balance indicators under the canopy of the forest as compared to the open area.

In this connection, active systematic studies of solar radiation started in 1924 in Feodosia (located next to the Karadag Nature Reserve) and transferred to Karadag in 1932 (Sivkov, 1932) mean that, despite being interrupted in some periods, there is a fairly large number of observations for the southeastern Crimea as a whole. There are also separate data on the night-time course of effective radiation on Karadag (Chumakova, 1947). Major studies of the radiation balance at Karadag were carried out by E.P. Barashkova (Barashkova, 1961). However, despite the large number of observations carried out from 1951–1956, they were carried out in an open area and can thus only be referred to indirectly. In general, these data cannot be used to provide a detailed characterisation of the values of the radiation balance and its elements in the forest ecosystems located within the Karadag Nature Reserve.

In the 1970s and 80s, separate work was carried out for the forests of the Crimean Peninsula to assess the radiation balance. However, their data cannot be compared with ours due to their being performed using completely different research methods and at different times. For example, I.P. Ved’ (Ved’, 1969) investigated the radiation balance of pine forests, but during the spring period. Ved’ (Ved’, 1974) also studied the radiation balance of pine forests located within the Crimean sub-Mediterranean region (near the city of Alushta). Here, data obtained in summer showed the radiation balance in the pine forest to be 314 kcal/cm2, while, in the open area, the corresponding figure was 241 kcal/cm2. In (Ved’, 1971) there are data on the radiation balance and its elements for beech forests, as well as for an open area located next to an oak forest.

There are various scattered data on the radiation balance and its elements for the territory of the Karadag Nature Reserve as a whole. A.A. Borisov (Borisov, 1963) provides the following annual data for Karadag: the value of the radiation balance is 53.2 kcal cm2; reflected radiation – 24.7 kcal/cm2; absorbed radiation – 95.3 kcal/cm2; effective radiation – 42.1 kcal/cm2.

The radiation balance of the territory as a whole depends both on the climatic parameters and the nature of the underlying surface. However, the main parameter on which the components of the radiation balance depend is the total influx of solar radiation. According to the values obtained by us, 5700 MJ/m2 enters the permanent study area annually, which corresponds to the values of the parameter under consideration in typical Mediterranean oak forests. Comparable regions of Italy and France are characterised by values of about 5400 MJ/m2, while for the downy-oak forests of Portugal, the corresponding values on average reach up to 6200 MJ/m2 (Baldocchi, 2010). Thus, the presented values support the conclusion that optimal environmental conditions for the growth of a downy-oak community have been formed in Crimea from the point of view of solar radiation. Comparison of the data obtained by us with similar ones for other territories is problematic, since in most works for Karadag, averaged values are given, potentially representing either greatly overestimated or underestimated data. For example, according to (Antyufeev, 1988), the values of the radiation balance at Karadag retain 2365 MJ/m2, which is much higher than the data we received in view of the regional scale of the study.

Another important unresolved issue is the underdeveloped character of balance studies in the study area. Although the weak development of such studies was already being noted by researchers of the second half of the twentieth century, it is still possible to reverse this trend.

However, a significant problem remains concerning the need to recalculate the data. In most previously published works (especially works published in the USSR prior to 1990), the data are given in kcal/cm2, which causes difficulties in their analysis and comparison with those obtained by us as a result of the study. In the works of some authors (Konstantinov, 1966; Shikhlinsky, 1969; Gvasalia, 1987), the dimensions of cal/cm2·min, kcal/cm2·month, kcal/cm2·year are also used to characterise the radiation balance and its components.

In the majority of radiation balance studies, the influence of crown relief is disregarded. In our opinion, this is an extremely serious omission. Due to the complex morphometry of the crown relief, the transmission of solar radiation by the crowns under the canopy of the forest is inhomogeneous. In addition to spatial differentiation, significant changes in the distribution of shadow masks over time, depending on the time of day, are also recorded. This phenomenon naturally depends on the height of the sun above the horizon, as well as its position, so that relatively open areas can be in the shade, while light will flow under the crowns of trees. Along with the described processes, however, the important role played by the growth characteristics of downy oak is also worth noting. The growth characteristics are understood in terms of the shape of trees and the main branches that form a wide crown. This type of forest is characterised by areas in which, being directly in the centre under the crown of a large tree, the branches form “windows” through which solar radiation can freely penetrate to the Earth’s surface. A similar situation is created by the mountainous terrain; here, the vertical distribution of vegetation along the slope forms gaps between branches of different levels, through which solar radiation enters. Therefore, an assessment of the considered downy-oak community only from the point of view of the closeness of the crowns would be incorrect. A typical example within the framework of the downy-oak community is given at point No. 3, in which there is a relatively small percentage of closure and density of crowns, but it is in the shade throughout the daylight, and point No. 12, in which a large amount of solar radiation penetrates to the Earth’s surface at 100% canopy closure.

Conclusions

As a result of the conducted research, general information was obtained on the inter-annual and intra-annual dynamics of incoming solar radiation in the downy-oak forests. The radiation balance of downy-oak forests for the period from 2010 to 2021 was calculated. Primary data on the distribution of total solar radiation over the vertical structure of downy-oak forests were obtained. It is shown that the flux of solar radiation onto the crown surface in clear windless weather is determined by the steepness of the crown relief and the orientation of the slope in relation to the sun within a specific period of time. The maximum values were observed on gentle slopes at 12:00. The spatiotemporal differentiation of the values of total solar radiation penetrating into the subsurface space within daylight hours, depending on the position and closeness of the crowns, is revealed.

Spatial models of transmission of aggregate solar radiation by the crowns of forests are constructed. A strong spatio-temporal heterogeneity in the transmission of total solar radiation by the canopy was revealed, which is no longer due to the closeness of the tree crowns, but rather to the density of the leaf overlap and the “windows” formed between the branches of trees. Within the limits of downy-oak forests, there is a large range of transmission values from 8 to 100%.

Elements of the short-wave part of the radiation balance of the crown surface and the long-wave part in the downy-oak forests were calculated. The average values of the elements of the short-wave part indicate the nature of the change with the maximum radiation values obtained at noon. The difference between the minimum and maximum values demonstrates the complexity of the crown relief of the studied types of forests, as well as the distribution of incoming radiation flows in them. Increasing values of long-wave radiation indicates a gradual warming of the soil cover throughout the day and the return of heat to the surface layer of air, which, when compared with the short-wave part, forms a negative radiation balance in the shaded areas of the under-canopy space and a positive balance in the open spaces. This distribution of values demonstrates the complex organisation and flow of the distribution of matter and energy fluxes in the downy-oak forest communities.

The barrier effect of the Crimean Mountains allowed the formation of a sub-Mediterranean type of climate on the southern macroslope, with the growth of downy oak forests. In this regard, the study of the radiation balance of forests located on the border of their range of existence is an extremely important task, including from the point of view of ecosystem and environment-forming functions, which make it possible to understand and reveal individual patterns of their internal organization.

This work was carried out within the framework of IBSS state research assignment “Studying the features of the functioning and dynamics of subtropical and tropical coastal ecosystems under the climate change and anthropogenic load using remote sensing, cloud information processing, and machine learning to create a scientific basis for their rational use” (No. 124030100030-0).

References

1. Budyko, M. I. (1961). The Heat Balance of the Earth’s Surface. Soviet Geography, 2(4), 3-13. https://doi.org/10.1080/00385417.1962.10769936
2. Berlyand, M.E. Prediction and regulation of the thermal regime of the surface layer of the atmosphere; Gidrometeoizdat: Leningrad, USSR, 1956; 435 p.
3. Budyko, M. I. Energy budget of the earth’s surface; Gidrometeoizdat: Leningrad, USSR, 1956; 286 p.
4. Reifsnyder, W. E. (1967). Forest meteorology: the forest energy balance. International Review of Forestry Research, 2, 127-179. https://doi.org/10.1016/B978-1-4831-9976-4.50010-8
5. Dzerdzeevskii, B. L. (1963). Study of the Heat Balance of the Forest. Silva Fennica, 113(7), 3-17.
6. Moore, C. J. (1976). A comparative study of radiation balance above forest and grassland. Quarterly Journal of the Royal Meteorological Society, 102(434), 889-899. https://doi.org/10.1002/qj.49710243416
7. Bergen, J. D. Nocturnal radiation loss estimates for a forest canopy. In Rocky Mountain Forest and Range Experiment Station; Forest Service: US Department of Agriculture, USA, 1969.
8. Lull, H. W.; Reigner, I. C. Radiation measurements by various instruments in the open and in the forest. In Northeastern Forest Experiment Station; Forest Service: US Department of Agriculture, USA, 1967.
9. Akimova, D. P. (1972). Radiation and thermal regimes in oak-pine crops at an altitude of 1300 m and in the field of nutrition of mineral sources. Scientific Works of the All-Russian Scientific Research Institute of Forestry and Forestry Mechanization, 11, 3-11.
10. Alekseev, V. A. The light regime of the forest. Nauka: Leningrad, USSR, 1975; pp. 1-227.
11. Vygodskaya, N.N. Methodology of phytoactinometric studies in mountainous areas. In Phytoactinometric studies of mountain forests; USSR Academy of Sciences: Vladivostok, USSR, 1977; pp. 20-37.
12. Vygodskaya, N. N. Radiation regime of 30-year-old oak forest in daily and seasonal dynamics. In Light regime, photosynthesis and forest productivity; Nauka: Moscow, USSR, 1967; pp. 77-94.
13. Zukert, N. V.; Vygodskaya, N. N.; Lebedeva, M. G.; Sherman, E. V. Radiation regime under the canopy of mountain forests. In Phytoactinometric studies of mountain forests; USSR Academy of Sciences: Vladivostok, USSR, 1967; pp. 162-178.
14. Bityukov, N. A. (2012). Radiation regime of parent beech stands of the Sochi National Park. Geographical research of the Krasnodar Territory: Collection of scientific papers, 7, 56-68.
15. Ugarov, I. S. (2018). The radiation budget of a forest in Central Yakutia. Science Almanac, 9-2(47), 128-133. https://doi.org/10.17117/na.2018.09.02.128
16. Korsakova, S. P. (2015). Agroclimatic resources of a lot № 32 in Nikitsky Botanical Gardens. Scientific Notes of the Nature Reserve «Cape Martyan», 6, 6-19.
17. Kondratyev, K. J.; Manolova, M. P. (1960). The radiation balance of slopes. Solar Energy, 4(1), 14-19.
18. Schaab, G.; Lenz, R.; Seufert, G. (1999). A temporal-spatial solar radiation model to improve scaling of biogenic emissions from a sparse Mediterranean pine/oak forest. Physics and Chemistry of the Earth Part B Hydrology Oceans and Atmosphere, 24(6), 673-680. https://doi.org/10.1016/S1464-1909(99)00064-7
19. Gorbunov, R. V.; Gorbunova, T. Yu.; Tabunshchik, V. A.; Drygval, A. V. (2020a). Structure and interannual dynamics of the regional ecosystems radiation balance of the plain Crimea. Ekosistemy, 23, 5–15. https://doi.org/10.37279/2414-4738-2020-23-5-15
20. Gorbunov, R. V.; Gorbunova, T. Yu.; Tabunshchik, V. A.; Drygval, A. V. (2020b). The radiation balance of oak forest ecosystems of the Crimean Peninsula. RUDN Journal of Ecology and Life Safety, 28(3), 201–212. http://dx.doi.org/10.22363/2313-2310-2020-28-3-201-212
21. Smirnov, V. O. (2016). Analysis of Geophysical Parameters of the Reserve «Cape Martyan»: Calculations of Direct, Diffuse and Total Radiation. Scientific Notes of the «Cape Martyan» Nature Reserve, 7, 56-61.
22. Smirnov, V. O. (2012). Some aspects of fitoaktinometrical research in the forests of reserve «Cape Martian». Geopolitics and Ecogeodynamics of regions, 8 (1-2), 104–109.
23. Ergina, E. I.; Gorbunov, R. V.; Tronza, G. E. (2017). Study and identification of soil standards and rare soils for the purpose of monitoring and protection of soil resources in the Plain Crimea; Arial: Simferopol, Russia
24. Garkusha, L. Y.; Bagrova, L.A. (2012). Composition, structure and modern environmental state of «Dubki» at the forest-steppe belt of the Crimea mountains. Optimization and Protection of Ecosystems, 6, 62–75
25. Antyufeev, V. V. (1988). Radiation Balance and Stability of Mountain-Forest Ecosystems in Recreation Areas of the Crimea. Proceedings of Nikitsky Botanical Garden, 104, 140-152.
26. Fursa, D. I.; Korsakova, S. P.; Amirdzhanov, A. G.; Fursa, V. P. Radiation and hydrothermal regime of the southern coast of Crimea according to the data of the agrometeorological station » Nikitsky Botanical Garden» for 1930-2004 and its consideration in the practice of viticulture; Nikitsky Botanical Garden: Yalta,Ukraine, 2006; 55 p.
27. Goisa, N. I. (1964). Radiation balance and effective radiation on the territory of Ukraine and Moldova. Proceedings of Ukrainian Hydrometeorological Institute, 41, 3-22.
28. Goisa, N. I. (1961). Distribution of total radiation over the territory of Ukraine and Moldova. Proceedings of Ukrainian Hydrometeorological Institute, 26, 14-28.
29. Borisov, A. A. (1963). Radiation regime of Crimea. Materialy po meteorologii i klimatologii, 1, 24-40.
30. Barashkova, E. P. Karadag radiation balance. In Actinometry and atmospheric optics. Hydrometeoizdat: Leningrad, USSR, 1961; pp. 42-47.
31. Chumakova, M. S. (1947). On the night course of effective radiation at Karadag. Proceedings of the Main Geophysical Observatory, 5, 25-31.
32. Sivkov, S. I. (1940). Some conclusions from actinometric observations in Feodosia and Karadag. Meteorology and Hydrology, 10, 3-13.
33. Ukrainian SSR. Atlas. Climatic Atlas of the Ukrainian SSR [Maps]; ed. by G. F. Prikhodko. Hydrometeoizdat: Leningrad, USSR, 1968; 232 p.
34. National Atlas of Ukraine; chief editor L. G. Rudenko; Chairman Editor Kol. B. E. Paton; K.: Cartography: Kiev, Ukraine, 2007; 435 p.
35. Song, Q. H.; Fei, X. H.; Zhang, Y. P.; Sha, L. Q.; Liu, Y. T.; Zhou, W. J.; Wu, C.-S.; Lu, Z.-Y.; Luo, K.; Gao J.-B.; Liu, Y. H. (2017). Water use efficiency in a primary subtropical evergreen forest in Southwest China. Scientific Reports, 7(1), 43031. https://doi.org/10.1038/srep43031
36. Wu, J.; Guan, D.; Han, S.; Shi, T.; Jin, C.; Pei, T.; Yu, G. (2007). Energy budget above a temperate mixed forest in northeastern China. Hydrological Processes: An International Journal, 21(18), 2425-2434. https://doi.org/10.1002/hyp.6395
37. Wang, X. C.; Liu, F.; Wang, C. K. (2022). Radiation and energy balance on a hillslope forest: horizontal versus slope-parallel installation of radiometer. Journal of Mountain Science, 19(11), 3076-3087. https://doi.org/10.1007/s11629-022-7481-8
38. Dudorova, N.  V.; Belan, B. D. (2015). Radiation balance of underlying surface in Tomsk during 2004–2005. Atmospheric and Oceanic Optics, 28(4), 312-317. https://doi.org/10.1134/S1024856015040065
39. Kharyutkina, E. V.; Ippolitov, I. I.; Loginov, S. V. (2012). The variability of radiative balance elements and air temperature over the Asian region of Russia. Biogeosciences, 9, 1113-1123. https://doi.org/10.5194/bg-9-1113-2012
40. Li, Z.; Deng, X.; Shi, Q.; Ke, X.; Liu, Y. (2013). Modeling the impacts of boreal deforestation on the near-surface temperature in European Russia. Advances in Meteorology, 2013, 486962. https://doi.org/10.1155/2013/486962
41. Parker, G.G.; Harmon, M.E.; Lefsky, M.A.; Chen, J.; Pelt, R.V.; Weis, S.B.; Thomas, S.C.; Winner, W.E.; Shaw, D.C.; Frankling, J.F. (2004). Three-dimensional structure of an old-growth pseudotsuga-tsuga canopy and its implications for radiation balance, microclimate, and gas exchange. Ecosystems, 7, 440–453. https://doi.org/10.1007/s10021-004-0136-5
42. Giambelluca, T. W.; Hölscher, D.; Bastos, T. X.; Frazão, R. R.; Nullet, M. A.; Ziegler, A. D. (1997). Observations of albedo and radiation balance over postforest land surfaces in the eastern Amazon basin. Journal of Climate, 10(5), 919-928. https://doi.org/10.1175/1520-0442(1997)010<0919:OOAARB>2.0.CO;2
43. Bastable, H. G.; Shuttleworth, W. J.; Dallarosa, R. L. G.; Fisch, G.; Nobre, C. A. (1993). Observations of climate, albedo, and surface radiation over cleared and undisturbed Amazonian forest. International Journal of Climatology, 13(7), 783-796. https://doi.org/10.1002/joc.3370130706
44. Giambelluca, T. W.; Fox, J.; Yarnasarn, S.; Onibutr, P.; Nullet, M. A. (1999). Dry-season radiation balance of land covers replacing forest in northern Thailand. Agricultural and Forest Meteorology, 95(1), 53-65. https://doi.org/10.1016/S0168-1923(99)00016-7
45. Ghimire, C. P.; van Meerveld, H. I.; Zwartendijk, B. W.; Bruijnzeel, L. A.; Ravelona, M.; Lahitiana, J.; Lubczynski, M. W. (2022). Vapour pressure deficit and solar radiation are the major drivers of transpiration in montane tropical secondary forests in eastern Madagascar. Agricultural and forest meteorology, 326, 109159. https://doi.org/10.1016/j.agrformet.2022.109159
46. Stanhill, G.; Israeli, M.; Rosenzweig, D. (1973). The solar radiation balance of scrub forest and pasture on the Carmel Mountain, Israel: a comparative study. Ecology, 54(4), 819-828. https://doi.org/10.2307/1935676
47. Seyednasrollah, B.; Kumar, M. (2019). How Surface Radiation on Forested Snowpack Changes across a Latitudinal Gradient. Hydrology, 6, 62; https://doi.org/10.3390/hydrology6030062.
48. Todt, M.; Rutter, N.; Fletcher, C. G.; Wake, L. M. (2019). Simulated single-layer forest canopies delay Northern Hemisphere snowmelt. The Cryosphere, 13(11), 3077-3091. https://doi.org/10.5194/tc-13-3077-2019
49. Fu, P.; Rich, P.M. (2002). A Geometric Solar Radiation Model with Applications in Agriculture and Forestry. Computers and Electronics in Agriculture, 37, 25–35. https://doi.org/10.1016/S0168-1699(02)00115-1
50. Isabelle, P. E.; Nadeau, D. F.; Asselin, M. H.; Harvey, R.; Musselman, K. N.; Rousseau, A. N.; Anctil, F. (2018). Solar radiation transmittance of a boreal balsam fir canopy: Spatiotemporal variability and impacts on growing season hydrology. Agricultural and forest meteorology, 263, 1-14. https://doi.org/10.1016/j.agrformet.2018.07.022
51. Schleppi, P.; Paquette, A. (2017). Solar Radiation in Forests: Theory for Hemispherical Photography. In: Hemispherical Photography in Forest Science: Theory, Methods, Applications. Managing Forest Ecosystems, Fournier, R., Hall, R. (eds); Springer: Dordrecht, Netherlands, 2017; Volume 28, pp. 15-52. https://doi.org/10.1007/978-94-024-1098-3_2
52. Terez E. I.; Terez G. A. (2002). Investigation of atmospheric transmission in the Crimea (Ukraine) in the twentieth century. Journal of applied meteorology, 41 (10), 1060-1063. https://doi.org/10.1175/1520-0450(2002)041<1060:IOATIT>2.0.CO;2
53. Webster, C.; Rutter, N.; Jonas, T. (2017). Improving representation of canopy temperatures for modeling subcanopy incoming longwave radiation to the snow surface. J. Geophys. Res. Atmos., 122, 9154–9172, https://doi.org/10.1002/2017JD026581.
54. Olpenda, A.S.; Sterenczak; K., Bedkowski, K. (2018). Modeling Solar Radiation in the Forest Using Remote Sensing Data: A Review of Approaches and Opportunities. Remote Sens., 10, 694; https://doi.org/10.3390/rs10050694
55. Simonson, W.; Allen, H.D.; Coomes, D. (2014). Overstorey and topographic effects on understories: Evidence for linkage from cork oak (Quercus suber) forests in Southern Spain. Forest Ecology and Management, 328, 35-44. https://doi.org/10.1016/j.foreco.2014.05.009
56. Yilmaz, O. Y.; Sevgi, O.; Koc, A. (2012). Determination of incoming solar radiation in major tree species in Turkey. Journal of Environmental Biology, 33(4), 817-821.
57. Liakatas, A.; Proutsos, N.; Alexandris, S. G. (2006). Optical properties affecting the radiant energy of an oak forest. Meteorological Application. Science and Technology for Weather and Climate, 9(4), 433-436. https://doi.org/10.1017/S135048270200405X
58. Bokov, V. (2001). Landscape — geophysical conditions of forests’ growing in Southeast Crimea; Tavriya-Plus: Simferopol, Ukraine, 2001;136 pp.
59. MERRA-2 meteorological re-analysis. Available online: URL: https://www.soda-pro.com/web-services/meteo-data/merra (accessed on 10 01 2023).
60. Rakhmanov, V.V. (1984). The hydroclimatic role of forests; Lesnaya promyshlennost’, Moscow, USSR, 1984; 240 p.
61. Brunt, D. (1932). Notes in Radiation in the Atmosphere. Quarterly Journal of the Royal Meteorological Society, 58, 389–418. http://dx.doi.org/10.1002/qj.49705824704
62. Gorbunov, R. V.; Gorbunova, T. Yu.; Kuznetsov, A. N.; Kuznetsova, S. P.; Lebedev, Ya. O.; Nguen, D. H.; Vu, M. (2019). Peculiarities of Formation of Radiation Balance Elements in the Mid-Mountain Tropical Forests of Southern Vietnam. Proceedings of the T.I.Vyazemsky Karadag Scientific Station — Nature Reserve of the Russian Academy of Sciences, 4(12), 3-16. https://doi.org/10.21072/eco.2021.12.01
63. Ved’, I. Р. (1969). Peculiarities of energy budget and radiation balance of pine plantations under frost conditions. Izvestiya USSR Academy of Sciences, Geography, 4, 109-113.
64. Ved’, I. Р. (1974). The Radiation Balance and the Phytoclimate of Young Stands of Pinus Pallasiana Lamb. Lesovedeniye, 5, 3-9.
65. Ved’, I. Р. (1971). Energy budget and radiation balance of the forest in the Crimean Highlands. Izvestiya USSR Academy of Sciences, Geography, 2, 61-70.
66. Baldocchi, D. D.; Ma, S.; Rambal, S.; Misson, L.; Ourcival, J.-M.; Limousin, J.-M.; Pereira, J.; Papale, D. (2010). On the differential advantages of evergreenness and deciduousness in mediterranean oak woodlands: a flux perspective. Ecological Applications, 20(6), 1583–1597. https://doi.org/10.1890/08-2047.1
67. Konstantinov, A. R.; Sakali, L. I.; Goysa, N. I.; Oleinik, R. N. Thermal and water regime of Ukraine; Hydrometeoizdat: Leningrad, USSR, 1966; 592 p.
68. Shikhlinsky, E.M. Energy budget of Azerbaijan SSR; Elm: Baku, USSR, 1969; 201 p.
69. Gvasalia, N. V. Energy budget of Georgia. Metsniereba: Tbilisi, UUSR, 1986; 116 p.

М. С. Сафонова1В. А. Табунщик Р. В. Горбунов Т. Ю. Горбунова	Радиационный баланс низкогорных субсредиземноморских ландшафтов (на примере Карадагского заповедника)
	ФГБУН Федеральный исследовательский центр «Институт биологии южных морей имени А.О. Ковалевского РАН», Севастополь, Россияe-mail:1malashina@ibss-ras.ru

Аннотация. Представлены новые количественные данные об элементах радиационного баланса пушистых дубовых лесов юго-восточного Крыма на примере Карадагского заповедника. Рассчитаны элементы коротковолновой части радиационного баланса поверхности крон и длинноволновой части в пушистых дубовых лесах. Впервые для лесов Юго-Восточного Крыма рассчитана величина поступления солнечной радиации на поверхность крон в пушистых дубовых и можжевеловых лесах совместно с величинами пропускания солнечной радиации под пологом леса. Построены пространственные модели пропускания суммарной солнечной радиации кронами пушистых дубовых лесов. Показано, что на величину пропускания солнечной радиации большое влияние оказывают форма и густота листьев, а также форма ветвей. Полученные данные показывают сложное пространственно-временное распределение солнечной радиации на поверхности крон и в подкроновом пространстве в пределах пушистых дубовых лесов.

Ключевые слова: пушистый дубовый лес; радиационный баланс; Карадаг; Крымский полуостров.

Литература

1. Будыко, М.И. Тепловой баланс поверхности Земли // Советская география. 1961. Т. 2, № 4. С. 3-13. DOI: 10.1080/00385417.1962.10769936
2. Берлянт, М.Е. Прогнозирование и регулирование теплового режима поверхностного слоя атмосферы. Л.: Гидрометеоиздат, 1956. 435 с.
3. Будыко, М.И. Энергетический бюджет поверхности Земли. Л.: Гидрометеоиздат, 1956. 286 с.
4. Reifsnyder, W. E. Forest meteorology: the forest energy balance // International Review of Forestry Research. 1967. Т. 2. С. 127-179. DOI: 10.1016/B978-1-4831-9976-4.50010-8
5. Дзердзевский, Б.Л. Исследование теплового баланса леса // Сила Фенника. 1963. Т. 113, № 7. С. 3-17.
6. Moore, C. J. A comparative study of radiation balance above forest and grassland // Quarterly Journal of the Royal Meteorological Society. 1976. Т. 102, № 434. С. 889-899. DOI: 10.1002/qj.49710243416
7. Bergen, J. D. Nocturnal radiation loss estimates for a forest canopy. In Rocky Mountain Forest and Range Experiment Station // В: Лесной экспериментальный станций Rocky Mountain. US Department of Agriculture, 1969.
8. Лалл, Х. В.; Рейгнер, И. К. Измерения радиации различными инструментами в открытом пространстве и в лесу // Forest Service: US Department of Agriculture, 1967.
9. Акимова, Д.П. Радиационные и тепловые режимы в дубово-сосновых насаждениях на высоте 1300 м и в зоне питания минеральных источников // Научные труды Всероссийского НИИ лесоводства и механизации лесного хозяйства. 1972. Т. 11. С. 3-11.
10. Алексéев, В.А. Световой режим леса. Л.: Наука, 1975. С. 1-227.
11. Выгодская, Н.Н. Методология фитоактинометрических исследований в горных районах // В: Фитоактинометрические исследования горных лесов. Владивосток: АН СССР, 1977. С. 20-37.
12. Выгодская, Н.Н. Радиационный режим 30-летнего дубового леса в суточной и сезонной динамике // В: Световой режим, фотосинтез и продуктивность леса. М.: Наука, 1967. С. 77-94.
13. Зукерт, Н.В., Выгодская, Н.Н., Лебедева, М.Г., Шерман, Е.В. Радиационный режим под пологом горных лесов // В: Фитоактинометрические исследования горных лесов. Владивосток: АН СССР, 1967. С. 162-178.
14. Битюков, Н.А. Радиационный режим родительских буковых насаждений Сочинского национального парка // Географические исследования Краснодарского края: Сборник научных работ. 2012. Т. 7. С. 56-68.
15. Угаров, И.С. Радиационный бюджет леса в Центральной Якутии // Научный альманах. 2018. № 9-2(47). С. 128-133. DOI: 10.17117/na.2018.09.02.128
16. Корсакова, С.П. Агроклиматические ресурсы участка № 32 в Никитском ботаническом саду // Научные записки заповедника «Мыс Мартьян». 2015. Т. 6. С. 6-19.
17. Кондратьев, К. И.; Манолова, М.П. Радиационный баланс склонов // Солнечная энергетика. 1960. Т. 4, № 1. С. 14-19.
18. Schaab, G.; Lenz, R.; Seufert, G. A temporal-spatial solar radiation model to improve scaling of biogenic emissions from a sparse Mediterranean pine/oak forest // Physics and Chemistry of the Earth Part B Hydrology Oceans and Atmosphere. 1999. Т. 24, № 6. С. 673-680. DOI: 10.1016/S1464-1909(99)00064-7
19. Горбунов, Р.В.; Горбунова, Т.Ю.; Табунщик, В.А.; Дрыгваль, А.В. Структура и межгодовая динамика радиационного баланса региональных экосистем равнинного Крыма // Экосистемы. 2020. Т. 23. С. 5-15. DOI: 10.37279/2414-4738-2020-23-5-15
20. Горбунов, Р.В.; Горбунова, Т.Ю.; Табунщик, В.А.; Дрыгваль, А.В. Радиационный баланс дубовых лесных экосистем Крымского полуострова // Вестник РУДН. Экология и безопасность жизнедеятельности. 2020. Т. 28, № 3. С. 201-212. DOI: 10.22363/2313-2310-2020-28-3-201-212
21. Смирнов, В.О. Анализ геофизических параметров заповедника «Мыс Мартьян»: Расчеты прямой, диффузной и общей радиации // Научные записки заповедника «Мыс Мартьян». 2016. Т. 7. С. 56-61.
22. Смирнов, В.О. Некоторые аспекты фитоактинометрических исследований в лесах заповедника «Мыс Мартьян» // Геополитика и экогеодинамика регионов. 2012. Т. 8, № 1-2. С. 104–109.
23. Ергина, Е.И.; Горбунов, Р.В.; Тронза, Г.Е. Исследование и идентификация почвенных стандартов и редких почв для мониторинга и охраны почвенных ресурсов в равнинном Крыму // Arial: Симферополь, Россия, 2017.
24. Гаркуша, Л.Ю.; Багрова, Л.А. Состав, структура и современное экологическое состояние «Дубков» лесостепной зоны Крымских гор // Оптимизация и защита экосистем. 2012. Т. 6. С. 62–75.
25. Антюфеев, В.В. Радиационный баланс и стабильность горно-лесных экосистем в рекреационных зонах Крыма // Труды Никитского ботанического сада. 1988. Т. 104. С. 140-152.
26. Фурса, Д.И., Корсакова, С.П., Амирджанов, А.Г., Фурса, В.П. Радиационный и гидротермический режим южного побережья Крыма по данным агрометеорологической станции «Никитский ботанический сад» за 1930-2004 гг. и его учет в практике виноградарства. Ялта: Никитский ботанический сад, 2006. 55 с.
27. Гойса, Н.И. Радиационный баланс и эффективная радиация на территории Украины и Молдовы // Труды Украинского гидрометеорологического института. 1964. Т. 41. С. 3-22.
28. Гойса, Н.И. Распределение общей радиации на территории Украины и Молдовы // Труды Украинского гидрометеорологического института. 1961. Т. 26. С. 14-28.
29. Борисов, А.А. Радиационный режим Крыма // Материалы по метеорологии и климатологии. 1963. Т. 1. С. 24-40.
30. Барашкова, Е.П. Радиационный баланс Карадага // В: Акбинометрия и атмосферная оптика. Л.: Гидрометеоиздат, 1961. С. 42-47.
31. Чумакова, М.С. О ночном ходе эффективной радиации на Карадаге // Труды Главной геофизической обсерватории. 1947. Т. 5. С. 25-31.
32. Сивков, С.И. Некоторые выводы из актинометрических наблюдений в Феодосии и Карадаге // Метеорология и гидрология. 1940. № 10. С. 3-13.
33. УССР. Атлас. Климатический атлас Украинской ССР [Карты]; ред. Г. Ф. Приходько. Л.: Гидрометеоиздат, 1968. 232 с.
34. Национальный атлас Украины; глав. ред. Л. Г. Руденко; председатель редколлегии Б. Е. Патон. Киев: Картография, 2007. 435 с.
35. Song, Q. H.; Fei, X. H.; Zhang, Y. P.; Sha, L. Q.; Liu, Y. T.; Zhou, W. J.; Wu, C.-S.; Lu, Z.-Y.; Luo, K.; Gao J.-B.; Liu, Y. H. Water use efficiency in a primary subtropical evergreen forest in Southwest China// Scientific Reports. 2017. Т. 7, № 1. Арт. 43031. DOI: 10.1038/srep43031
36. Wu, J.; Guan, D.; Han, S.; Shi, T.; Jin, C.; Pei, T.; Yu, G. (2007). Energy budget above a temperate mixed forest in northeastern China // Hydrological Processes: An International Journal. 2007. Т. 21, № 18. С. 2425-2434. DOI: 10.1002/hyp.6395
37. Wang, X. C.; Liu, F.; Wang, C. K. (2022). Radiation and energy balance on a hillslope forest: horizontal versus slope-parallel installation of radiometer // Journal of Mountain Science. 2022. Т. 19, № 11. С. 3076-3087. DOI: 10.1007/s11629-022-7481-8
38. Дудорова, Н.В.; Белан, Б.Д. Радиационный баланс подстилающей поверхности в Томске за 2004–2005 гг. // Атмосферная и океаническая оптика. 2015. Т. 28, № 4. С. 312-317. DOI: 10.1134/S1024856015040065
39. Харютина, Е.В.; Ипполитов, И.И.; Логинов, С.В. Переменность элементов радиационного баланса и температуры воздуха в азиатском регионе России // Биогеонауки. 2012. Т. 9. С. 1113-1123. DOI: 10.5194/bg-9-1113-2012
40. Li, Z.; Deng, X.; Shi, Q.; Ke, X.; Liu, Y. (2013). Modeling the impacts of boreal deforestation on the near-surface temperature in European Russia // Advances in Meteorology. 2013. Арт. 486962. DOI: 10.1155/2013/486962
41. Parker, G.G.; Harmon, M.E.; Lefsky, M.A.; Chen, J.; Pelt, R.V.; Weis, S.B.; Thomas, S.C.; Winner, W.E.; Shaw, D.C.; Frankling, J.F. Three-dimensional structure of an old-growth pseudotsuga-tsuga canopy and its implications for radiation balance, microclimate, and gas exchange// Ecosystems. 2004. Т. 7. С. 440–453. DOI: 10.1007/s10021-004-0136-5
42. Giambelluca, T. W.; Hölscher, D.; Bastos, T. X.; Frazão, R. R.; Nullet, M. A.; Ziegler, A. D. Observations of albedo and radiation balance over postforest land surfaces in the eastern Amazon basin // Journal of Climate. 1997. Vol. 10, No. 5. P. 919-928. https://doi.org/10.1175/1520-0442(1997)0102.0.CO;2
43. Bastable, H. G.; Shuttleworth, W. J.; Dallarosa, R. L. G.; Fisch, G.; Nobre, C. A. Observations of climate, albedo, and surface radiation over cleared and undisturbed Amazonian forest // International Journal of Climatology. 1993. Vol. 13, No. 7. P. 783-796. https://doi.org/10.1002/joc.3370130706
44. Giambelluca, T. W.; Fox, J.; Yarnasarn, S.; Onibutr, P.; Nullet, M. A. Dry-season radiation balance of land covers replacing forest in northern Thailand // Agricultural and Forest Meteorology. 1999. Vol. 95, No. 1. P. 53-65. https://doi.org/10.1016/S0168-1923(99)00016-7
45. Ghimire, C. P.; van Meerveld, H. I.; Zwartendijk, B. W.; Bruijnzeel, L. A.; Ravelona, M.; Lahitiana, J.; Lubczynski, M. W. Vapour pressure deficit and solar radiation are the major drivers of transpiration in montane tropical secondary forests in eastern Madagascar // Agricultural and Forest Meteorology. 2022. Vol. 326. Art. 109159. https://doi.org/10.1016/j.agrformet.2022.109159
46. Stanhill, G.; Israeli, M.; Rosenzweig, D. The solar radiation balance of scrub forest and pasture on the Carmel Mountain, Israel: a comparative study // Ecology. 1973. Vol. 54, No. 4. P. 819-828. https://doi.org/10.2307/1935676
47. Seyednasrollah, B.; Kumar, M. How Surface Radiation on Forested Snowpack Changes across a Latitudinal Gradient // Hydrology. 2019. Vol. 6. P. 62. https://doi.org/10.3390/hydrology6030062
48. Todt, M.; Rutter, N.; Fletcher, C. G.; Wake, L. M. Simulated single-layer forest canopies delay Northern Hemisphere snowmelt // The Cryosphere. 2019. Vol. 13, No. 11. P. 3077-3091. https://doi.org/10.5194/tc-13-3077-2019
49. Fu, P.; Rich, P.M. A Geometric Solar Radiation Model with Applications in Agriculture and Forestry // Computers and Electronics in Agriculture. 2002. Vol. 37. P. 25–35. https://doi.org/10.1016/S0168-1699(02)00115-1
50. Isabelle, P. E.; Nadeau, D. F.; Asselin, M. H.; Harvey, R.; Musselman, K. N.; Rousseau, A. N.; Anctil, F. Solar radiation transmittance of a boreal balsam fir canopy: Spatiotemporal variability and impacts on growing season hydrology // Agricultural and Forest Meteorology. 2018. Vol. 263. P. 1-14. https://doi.org/10.1016/j.agrformet.2018.07.022
51. Schleppi, P.; Paquette, A. Solar Radiation in Forests: Theory for Hemispherical Photography // In: Hemispherical Photography in Forest Science: Theory, Methods, Applications. Managing Forest Ecosystems, Fournier, R., Hall, R. (eds). Dordrecht: Springer, 2017. Vol. 28. P. 15-52. https://doi.org/10.1007/978-94-024-1098-3_2
52. Terez, E. I.; Terez, G. A. Investigation of atmospheric transmission in the Crimea (Ukraine) in the twentieth century // Journal of Applied Meteorology. 2002. Vol. 41, No. 10. P. 1060-1063. https://doi.org/10.1175/1520-0450(2002)0412.0.CO;2
53. Webster, C.; Rutter, N.; Jonas, T. Improving representation of canopy temperatures for modeling subcanopy incoming longwave radiation to the snow surface // J. Geophys. Res. Atmos. 2017. Vol. 122. P. 9154–9172. https://doi.org/10.1002/2017JD026581
54. Olpenda, A.S.; Sterenczak, K.; Bedkowski, K. Modeling Solar Radiation in the Forest Using Remote Sensing Data: A Review of Approaches and Opportunities // Remote Sens. 2018. Vol. 10. Art. 694. https://doi.org/10.3390/rs10050694
55. Simonson, W.; Allen, H.D.; Coomes, D. Overstorey and topographic effects on understories: Evidence for linkage from cork oak (Quercus suber) forests in Southern Spain // Forest Ecology and Management. 2014. Vol. 328. P. 35-44. https://doi.org/10.1016/j.foreco.2014.05.009
56. Yilmaz, O. Y.; Sevgi, O.; Koc, A. Determination of incoming solar radiation in major tree species in Turkey // Journal of Environmental Biology. 2012. Vol. 33, No. 4. P. 817-821.
57. Liakatas, A.; Proutsos, N.; Alexandris, S. G. Optical properties affecting the radiant energy of an oak forest. Meteorological Application // Science and Technology for Weather and Climate. 2006. Т. 9, № 4. С. 433-436. DOI: 10.1017/S135048270200405X
58. Боков, В. Ландшафтно-геофизические условия роста лесов в Юго-восточном Крыму. Симферополь: Таврия-Плюс, 2001. 136 с.
59. MERRA-2. Метеорологический реанализ. Доступно онлайн: URL: https://www.soda-pro.com/web-services/meteo-data/merra (дата доступа: 01.10.2023).
60. Рахманов, В.В. Гидроклиматическая роль лесов. М.: Лесная промышленность, 1984. 240 с.
61. Брант, Д. Замечания о радиации в атмосфере // Квартальный журнал Королевского метеорологического общества. 1932. Т. 58. С. 389–418. DOI: 10.1002/qj.49705824704
62. Горбунов, Р. В.; Горбунова, Т. Ю.; Kuznetsov, A. N.; Кузнецова, С. П.; Лебедев, Я. О.; Нгуен, Д. Х.; Ву, М. Специфика формирования элементов радиационного баланса в среднем горном тропическом лесу Южного Вьетнама // Труды Карадагской научной станции им. Т.И. Вяземского — заповедника РАН. 2019. Т. 4, № 12. С. 3-16. DOI: 10.21072/eco.2021.12.01
63. Ведь, И. Р. Особенности энергетического бюджета и радиационного баланса сосняков в условиях морозов // Известия АН СССР. География. 1969. № 4. С. 109-113.
64. Ведь, И. Р. Радиативный баланс и фитоклимат молодняка сосны Палласа // Лесоведение. 1974. Т. 5. С. 3-9.
65. Ведь, И. Р. Энергетический бюджет и радиационный баланс леса в Крымских горах // Известия АН СССР. География. 1971. № 2. С. 61-70.
66. Baldocchi, D. D.; Ma, S.; Rambal, S.; Misson, L.; Ourcival, J.-M.; Limousin, J.-M.; Pereira, J.; Papale, D. On the differential advantages of evergreenness and deciduousness in mediterranean oak woodlands: a flux perspective // Ecological Applications. 2010. Т. 20, № 6. С. 1583–1597. DOI: 10.1890/08-2047.1
67. Константинов, А. Р.; Сакали, Л. И.; Гойса, Н. И.; Олейник, Р. Н. Термальный и водный режим Украины. Л.: Гидрометеоиздат, 1966. 592 с.
68. Shikhlinsky, E.M. Energy budget of Azerbaijan SSR; Elm: Baku, USSR, 1969; 201 p.
69. Gvasalia, N. V. Energy budget of Georgia. Metsniereba: Tbilisi, UUSR, 1986; 116 p.

Поступила в редакцию 10.09.2024 г.